Frame of reference: Difference between revisions
mNo edit summary |
mNo edit summary |
||
| Line 7: | Line 7: | ||
* Define frame of reference, and notation. Use u,v,w and x,y, and z? | * Define frame of reference, and notation. Use u,v,w and x,y, and z? | ||
* A frame of reference is a coordinate system whose origin and orientation are specified by a set of reference points. | * A frame of reference is a coordinate system whose origin and orientation are specified by a set of reference points. | ||
* Add sketch for shear probe? | |||
==Velocity measurement frame== | |||
The velocities are measured in either the instrument's coordinate system (XYZ), beam coordinates, or more rarely in the earth's coordinate system (ENU=east, north and up). When estimating <math>\varepsilon</math>, measured velocities are often rotated in the flow's frame of reference since [[Velocity inertial subrange model|inertial subrange model]] differs between the velocity components, and the effects of [[Anisotropic turbulence|anisotropy]] are more pronounced in the transverse and vertical direction. When the vertical velocities are too heavily impacted by [[Anisotropic turbulence|anisotropy]], the longitudinal direction may be used to derive <math>\varepsilon</math>. In these instances, the longitudinal velocity must align with the mean flow direction, which may require rotating the measurements into a new frame of reference i.e., the analysis frame of reference. | The velocities are measured in either the instrument's coordinate system (XYZ), beam coordinates, or more rarely in the earth's coordinate system (ENU=east, north and up). When estimating <math>\varepsilon</math>, measured velocities are often rotated in the flow's frame of reference since [[Velocity inertial subrange model|inertial subrange model]] differs between the velocity components, and the effects of [[Anisotropic turbulence|anisotropy]] are more pronounced in the transverse and vertical direction. When the vertical velocities are too heavily impacted by [[Anisotropic turbulence|anisotropy]], the longitudinal direction may be used to derive <math>\varepsilon</math>. In these instances, the longitudinal velocity must align with the mean flow direction, which may require rotating the measurements into a new frame of reference i.e., the analysis frame of reference. | ||
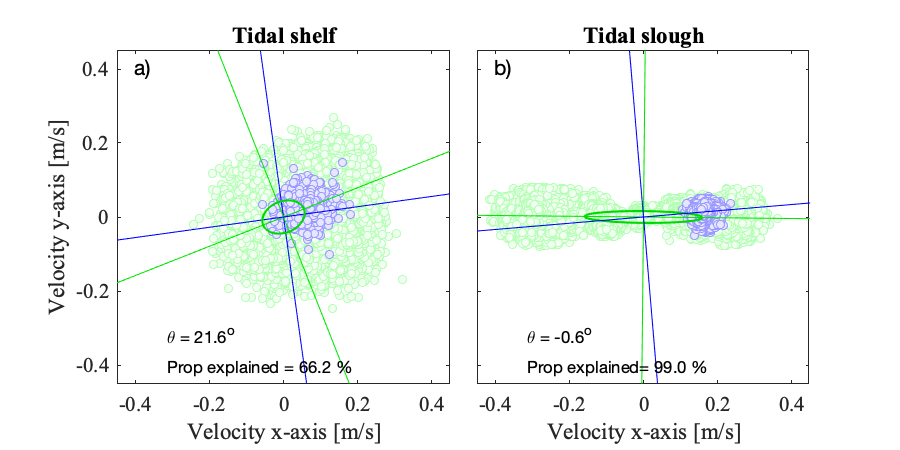
[[File:Frame of reference adv.png|600px|frame|center|Fig 1. Examples of measured velocities from two ADVs highlighting that their x-axis may be un-aligned with the flow's. The blue dots and lines that represent the principal axes are for a 5-min subset of the entire timeseries shown in green. The left example (a) is from the shelf break in ~190 m of water, while the right example (b) is from a tidally-influenced slough (a few meters deep). The statistics in each panel were estimated for the entire timeseries, and highlights that the flow's frame of reference is much more variable in the Tidal shelf example (a) than the Tidal Slough (b). Another key difference is that in the slough example (b), the instrument's x-axis was oriented along the length of the channel, and thus in the general direction of the flow. This is the ideal set-up for turbulence measurements from acoustic-Doppler velocity-meters.]] | [[File:Frame of reference adv.png|600px|frame|center|Fig 1. Examples of measured velocities from two ADVs highlighting that their x-axis may be un-aligned with the flow's. The blue dots and lines that represent the principal axes are for a 5-min subset of the entire timeseries shown in green. The left example (a) is from the shelf break in ~190 m of water, while the right example (b) is from a tidally-influenced slough (a few meters deep). The statistics in each panel were estimated for the entire timeseries, and highlights that the flow's frame of reference is much more variable in the Tidal shelf example (a) than the Tidal Slough (b). Another key difference is that in the slough example (b), the instrument's x-axis was oriented along the length of the channel, and thus in the general direction of the flow. This is the ideal set-up for turbulence measurements from acoustic-Doppler velocity-meters.]] | ||
<br> | <br> | ||
Revision as of 15:34, 2 June 2022
| Short definition of Frame of reference |
|---|
| A frame of reference is a coordinate system used to align the 3D velocity components |
This is the common definition for Frame of reference, but other definitions maybe discussed within the wiki.
In progress:
- Define frame of reference, and notation. Use u,v,w and x,y, and z?
- A frame of reference is a coordinate system whose origin and orientation are specified by a set of reference points.
- Add sketch for shear probe?
Velocity measurement frame
The velocities are measured in either the instrument's coordinate system (XYZ), beam coordinates, or more rarely in the earth's coordinate system (ENU=east, north and up). When estimating [math]\displaystyle{ \varepsilon }[/math], measured velocities are often rotated in the flow's frame of reference since inertial subrange model differs between the velocity components, and the effects of anisotropy are more pronounced in the transverse and vertical direction. When the vertical velocities are too heavily impacted by anisotropy, the longitudinal direction may be used to derive [math]\displaystyle{ \varepsilon }[/math]. In these instances, the longitudinal velocity must align with the mean flow direction, which may require rotating the measurements into a new frame of reference i.e., the analysis frame of reference.